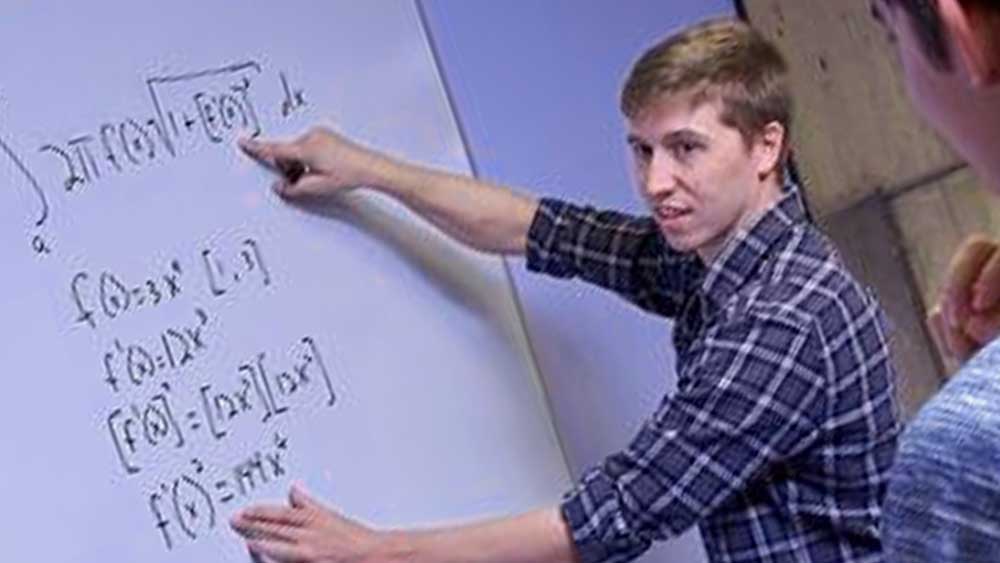Table of Contents
Mathematics is a fascinating subject that has been studied for thousands of years. It is a fundamental tool that is used in many fields, from science and engineering to finance and art.
At its core, mathematics is the study of numbers, shapes, and patterns. It involves the use of logical reasoning and problem-solving skills to arrive at solutions to a wide range of problems.
One of the earliest branches of mathematics is arithmetic, which deals with the basics of addition, subtraction, multiplication, and division. This is the foundation upon which more advanced mathematical concepts are built.
Geometry, another early branch of mathematics, is the study of shapes and spatial relationships. It involves the use of geometrical concepts such as points, lines, angles, and curves to solve problems and understand the properties of different shapes.
Calculus, on the other hand, is a branch of mathematics that deals with rates of change and the concept of limits. It is used to study the behavior of functions and to solve problems involving rates of change, such as finding the slope of a line or the area under a curve.
Algebra, another important branch of mathematics, is the study of equations and the relationships between variables. It is used to solve problems involving unknown values, and is an essential tool in many fields, such as engineering, economics, and finance.
Geometry
Geometry is a branch of mathematics that deals with the study of shapes and spatial relationships. It is a fundamental part of our understanding of the world around us, and has been studied for thousands of years.
Geometry originated in ancient Egypt, where it was used to measure land and to build structures such as pyramids. Later, in ancient Greece, the mathematician Euclid developed a systematic approach to geometry that is still used today.
Geometry involves the use of geometric concepts such as points, lines, angles, and curves to solve problems and understand the properties of different shapes. It also involves the use of logical reasoning and problem-solving skills to arrive at solutions to a wide range of problems.
One of the most important branches of geometry is Euclidean geometry, which is named after the mathematician Euclid. This branch of geometry deals with the properties of shapes in two-dimensional space, and includes the study of points, lines, angles, triangles, circles, and other geometric figures.
Another important branch of geometry is non-Euclidean geometry, which is a type of geometry that differs from Euclidean geometry in its assumptions about the nature of space. Non-Euclidean geometry is used to study the properties of shapes in three-dimensional space, and is used in fields such as physics and engineering.
In addition to these core branches of geometry, there are many other sub-disciplines that focus on specific areas of study. For example, topology is the study of shapes and their properties, while trigonometry is the study of triangles and their relationships with other geometric figures.
Despite its many complexities, geometry is a subject that can be studied and understood by anyone with a basic foundation in arithmetic and logical reasoning. With practice and dedication, anyone can learn to think like a geometer and use the power of geometry to solve a wide range of problems.
In conclusion, geometry is a fascinating and versatile branch of mathematics that is essential to our understanding of the world around us. Whether you are a scientist, engineer, or simply someone who enjoys solving puzzles, there is a place for geometry in your life.
Calculus
Calculus is a branch of mathematics that deals with the concept of change and the study of rates of change. It is a fundamental tool used in many fields, from science and engineering to economics and finance.
Calculus was developed independently by two mathematicians, Isaac Newton and Gottfried Leibniz, in the late 17th century. They were both trying to solve problems related to the motion of objects and the behavior of physical systems, and their work laid the foundation for the modern study of calculus.
Calculus involves the use of mathematical concepts such as limits, derivatives, and integrals to solve problems and understand the behavior of functions. It is used to study the rates of change of quantities, such as the slope of a line or the rate of change of velocity, and to find the areas under or between curves.
There are two main branches of calculus: differential calculus and integral calculus. Differential calculus is concerned with the study of rates of change and the concept of derivatives, while integral calculus is concerned with the concept of integration and the calculation of areas and volumes.
Calculus has many practical applications in fields such as science, engineering, and economics. In physics, calculus is used to study the motion of objects and the behavior of physical systems, such as the motion of planets or the flow of fluids. In engineering, calculus is used to design and analyze structures and systems, such as bridges and electrical circuits. In economics, calculus is used to study the behavior of markets and to make predictions about supply and demand.
Despite its many complexities, calculus is a subject that can be studied and understood by anyone with a strong foundation in algebra and geometry. With practice and dedication, anyone can learn to think like a mathematician and use the power of calculus to solve a wide range of problems.
In conclusion, calculus is a fascinating and versatile branch of mathematics that is essential to many fields of study. From its origins in the work of Newton and Leibniz, it has grown into a vast and complex field with many applications and sub-disciplines. Whether you are a scientist, engineer, or simply someone who enjoys solving puzzles, there is a place for calculus in your life.
Algebra
Algebra is a branch of mathematics that deals with the study of equations and the relationships between variables. It is a fundamental tool used in many fields, from science and engineering to finance and economics.
Algebra originated in ancient Babylonia and Egypt, where it was used to solve problems involving unknown quantities. Later, in ancient Greece, the mathematician Diophantus developed a systematic approach to algebra that is still used today.
Algebra involves the use of mathematical symbols and operations to represent and solve problems involving unknown values. It is used to study the relationships between different variables, and to solve equations and find solutions to a wide range of problems.
One of the most important branches of algebra is linear algebra, which is the study of linear equations and their solutions. Linear algebra is used to solve systems of equations and to understand the properties of vectors and matrices.
Another important branch of algebra is abstract algebra, which is the study of algebraic structures and their properties. Abstract algebra is used to study the properties of numbers, sets, and other algebraic objects, and is an essential tool in many fields, such as computer science and cryptography.
In addition to these core branches of algebra, there are many other sub-disciplines that focus on specific areas of study. For example, number theory is the study of numbers and their properties, while algebraic geometry is the study of geometric objects using the methods of algebra.
Despite its many complexities, algebra is a subject that can be studied and understood by anyone with a strong foundation in arithmetic and logical reasoning. With practice and dedication, anyone can learn to think like an algebraist and use the power of algebra to solve a wide range of problems.
In conclusion, algebra is a fascinating and versatile branch of mathematics that is essential to many fields of study. From its origins in ancient Babylonia and Egypt, it has grown into a vast and complex field with many applications and sub-disciplines. Whether you are a scientist, engineer, or simply someone who enjoys solving puzzles, there is a place for algebra in your life.
In addition to these core branches of mathematics, there are many other sub-disciplines that focus on specific areas of study. For example, number theory is the study of numbers and their properties, while combinatorics is the study of counting and arranging objects.
Despite its many complexities, mathematics is a subject that can be studied and understood by anyone with a basic foundation in arithmetic and logical reasoning. With practice and dedication, anyone can learn to think like a mathematician and use the power of mathematics to solve a wide range of problems.
In conclusion, mathematics is a fascinating and versatile subject that is essential to many fields of study. From its humble beginnings in arithmetic and geometry, it has grown into a vast and complex field with many sub-disciplines and applications. Whether you are a scientist, engineer, or simply someone who enjoys solving puzzles, there is a place for mathematics in your life.